Zodiac Compatibility
(See an interesting phenomenon that you would like to learn more about.) A friend of mine told me that he thought a pair of people's zodiac signs were a significant factor in whether or not the two people would get along well together. I found this to be intriguing, and so I decided to conduct an experiment to determine whether there might be weight to it.
(Develop a theory about how the phenomenon works, and then select a testable hypothesis about the phenomenon.) In this case, I'm not particularly interested in how it works, rather I'm am merely trying to determine whether it works at all [1]. Our null hypothesis is that there is no relationship between the likelihood that two people are friends based on their zodiac sign. If we can reject this null hypothesis, then there may be a basis of thinking that this particular aspect of astrology has some weight.
[1]Because empirical science is messier than analytical science, I'm not going to be quite as rigorous about the experiment that I conduct here. It's intention, after all, is to help illustrate the process, not meaningfully contribute to the state of the knowledge of objective reality.
(Determine exactly what evidence would need be gathered in order to test the hypothesis.) Since zodiac sign is a function of one's birthday, what's required is the birthdays of a given test subject, and all of their friends. Specifically, I will be using myself as the test subject, and Facebook as the source [2] for the birthdays of my friends. I will determine my friend's zodiac signs from their birthdays, then bucket them by their zodiac sign, and determine the extent to which this distribution deviates from the expected null-hypothesis distribution.
[2]This experimental setup introduces some additional assumptions into our hypothesis, like that there's no correlation between zodiac sign and whether one opts out of making one's birthday visible on Facebook to friends. Good empiricism always requires paying attention to the additional constraints.
(Determine the power level of the experiment, which is the strength of the effect you'd need to see in order to be satisfied that your null hypothesis must be false.) I'm going to be unfortunate and go ahead and blindly grab p=0.05. What this means is that I am satisfied with rejecting the null hypothesis if a more extreme result than the one observed from the data has a less than 5% chance of occurring. I have no good reason for choosing 0.05 in particular; there's no money or lives resting on the result that might warrant a higher level of doubt.
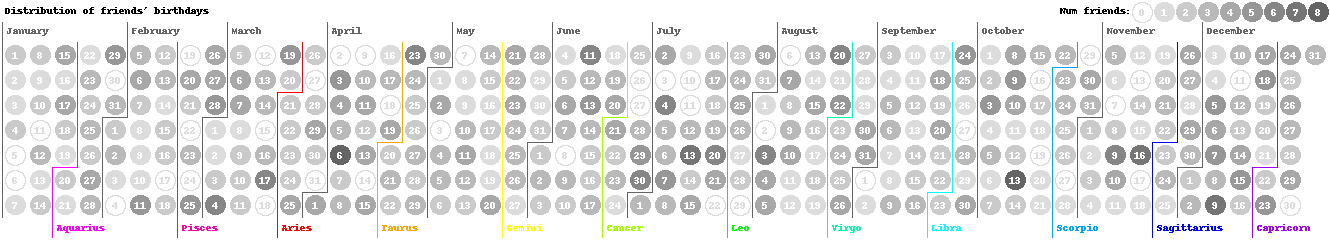
(Collect your data in a means that prevents you as the experimenter from subtley messing with the results.) Here's the list [3] of all my Facebook friends' visible birthdays (n=876, in (month, day) pairs):
[3]Most studies don't publish their raw data like this one does, which makes independent verification difficult.
| (1, 1) | (1, 1) | (1, 2) | (1, 3) | (1, 4) | (1, 4) | (1, 7) | (1, 8) | (1, 8) | (1, 9) | |
| (1, 10) | (1, 10) | (1, 12) | (1, 12) | (1, 12) | (1, 13) | (1, 14) | (1, 14) | (1, 15) | (1, 15) | |
| (1, 15) | (1, 16) | (1, 17) | (1, 17) | (1, 17) | (1, 17) | (1, 18) | (1, 18) | (1, 19) | (1, 20) | |
| (1, 20) | (1, 21) | (1, 22) | (1, 23) | (1, 23) | (1, 23) | (1, 24) | (1, 24) | (1, 25) | (1, 25) | |
| (1, 25) | (1, 26) | (1, 26) | (1, 27) | (1, 27) | (1, 27) | (1, 27) | (1, 28) | (1, 28) | (1, 28) | |
| (1, 29) | (1, 29) | (1, 29) | (1, 29) | (1, 29) | (1, 31) | (1, 31) | (1, 31) | (2, 1) | (2, 1) | |
| (2, 2) | (2, 2) | (2, 2) | (2, 3) | (2, 5) | (2, 5) | (2, 5) | (2, 6) | (2, 6) | (2, 6) | |
| (2, 6) | (2, 7) | (2, 7) | (2, 8) | (2, 9) | (2, 10) | (2, 11) | (2, 11) | (2, 11) | (2, 11) | |
| (2, 11) | (2, 12) | (2, 12) | (2, 13) | (2, 13) | (2, 13) | (2, 14) | (2, 15) | (2, 15) | (2, 16) | |
| (2, 16) | (2, 18) | (2, 18) | (2, 20) | (2, 20) | (2, 20) | (2, 20) | (2, 21) | (2, 21) | (2, 23) | |
| (2, 23) | (2, 23) | (2, 25) | (2, 25) | (2, 25) | (2, 25) | (2, 25) | (2, 26) | (2, 26) | (2, 26) | |
| (2, 26) | (2, 27) | (2, 27) | (2, 27) | (2, 27) | (2, 28) | (2, 28) | (2, 28) | (2, 28) | (2, 28) | |
| (3, 1) | (3, 2) | (3, 3) | (3, 3) | (3, 4) | (3, 4) | (3, 4) | (3, 4) | (3, 4) | (3, 4) | |
| (3, 5) | (3, 5) | (3, 6) | (3, 6) | (3, 6) | (3, 6) | (3, 7) | (3, 7) | (3, 7) | (3, 7) | |
| (3, 8) | (3, 9) | (3, 9) | (3, 10) | (3, 10) | (3, 11) | (3, 11) | (3, 13) | (3, 13) | (3, 13) | |
| (3, 14) | (3, 14) | (3, 14) | (3, 16) | (3, 16) | (3, 16) | (3, 17) | (3, 17) | (3, 17) | (3, 17) | |
| (3, 17) | (3, 17) | (3, 19) | (3, 19) | (3, 19) | (3, 19) | (3, 19) | (3, 20) | (3, 20) | (3, 21) | |
| (3, 21) | (3, 22) | (3, 22) | (3, 23) | (3, 23) | (3, 24) | (3, 24) | (3, 25) | (3, 25) | (3, 25) | |
| (3, 25) | (3, 26) | (3, 26) | (3, 28) | (3, 28) | (3, 29) | (3, 29) | (3, 29) | (3, 29) | (3, 30) | |
| (3, 30) | (4, 1) | (4, 1) | (4, 1) | (4, 1) | (4, 3) | (4, 3) | (4, 3) | (4, 3) | (4, 3) | |
| (4, 4) | (4, 4) | (4, 4) | (4, 4) | (4, 5) | (4, 5) | (4, 5) | (4, 6) | (4, 6) | (4, 6) | |
| (4, 6) | (4, 6) | (4, 6) | (4, 6) | (4, 6) | (4, 7) | (4, 7) | (4, 8) | (4, 8) | (4, 8) | |
| (4, 10) | (4, 10) | (4, 10) | (4, 11) | (4, 11) | (4, 11) | (4, 11) | (4, 12) | (4, 12) | (4, 13) | |
| (4, 13) | (4, 13) | (4, 13) | (4, 15) | (4, 15) | (4, 15) | (4, 15) | (4, 16) | (4, 17) | (4, 17) | |
| (4, 17) | (4, 19) | (4, 19) | (4, 19) | (4, 19) | (4, 20) | (4, 20) | (4, 21) | (4, 21) | (4, 21) | |
| (4, 22) | (4, 22) | (4, 23) | (4, 23) | (4, 23) | (4, 23) | (4, 23) | (4, 23) | (4, 24) | (4, 24) | |
| (4, 24) | (4, 25) | (4, 25) | (4, 26) | (4, 26) | (4, 27) | (4, 27) | (4, 27) | (4, 28) | (4, 28) | |
| (4, 29) | (4, 29) | (4, 29) | (4, 30) | (4, 30) | (4, 30) | (5, 1) | (5, 2) | (5, 2) | (5, 2) | |
| (5, 2) | (5, 4) | (5, 4) | (5, 4) | (5, 5) | (5, 5) | (5, 5) | (5, 6) | (5, 6) | (5, 8) | |
| (5, 9) | (5, 10) | (5, 10) | (5, 10) | (5, 11) | (5, 11) | (5, 11) | (5, 11) | (5, 12) | (5, 12) | |
| (5, 12) | (5, 13) | (5, 13) | (5, 14) | (5, 14) | (5, 14) | (5, 15) | (5, 15) | (5, 16) | (5, 17) | |
| (5, 17) | (5, 18) | (5, 19) | (5, 20) | (5, 20) | (5, 20) | (5, 20) | (5, 21) | (5, 21) | (5, 21) | |
| (5, 21) | (5, 22) | (5, 22) | (5, 22) | (5, 23) | (5, 23) | (5, 23) | (5, 23) | (5, 24) | (5, 24) | |
| (5, 24) | (5, 25) | (5, 25) | (5, 25) | (5, 26) | (5, 26) | (5, 26) | (5, 27) | (5, 28) | (5, 28) | |
| (5, 28) | (5, 29) | (5, 30) | (5, 31) | (5, 31) | (6, 1) | (6, 1) | (6, 2) | (6, 2) | (6, 2) | |
| (6, 3) | (6, 3) | (6, 4) | (6, 5) | (6, 5) | (6, 6) | (6, 6) | (6, 6) | (6, 6) | (6, 7) | |
| (6, 7) | (6, 7) | (6, 9) | (6, 9) | (6, 9) | (6, 10) | (6, 10) | (6, 11) | (6, 11) | (6, 11) | |
| (6, 11) | (6, 11) | (6, 11) | (6, 12) | (6, 12) | (6, 12) | (6, 13) | (6, 13) | (6, 13) | (6, 13) | |
| (6, 13) | (6, 14) | (6, 14) | (6, 14) | (6, 15) | (6, 15) | (6, 16) | (6, 16) | (6, 17) | (6, 17) | |
| (6, 17) | (6, 18) | (6, 19) | (6, 19) | (6, 19) | (6, 20) | (6, 20) | (6, 20) | (6, 20) | (6, 21) | |
| (6, 21) | (6, 21) | (6, 21) | (6, 22) | (6, 22) | (6, 23) | (6, 23) | (6, 23) | (6, 24) | (6, 25) | |
| (6, 25) | (6, 28) | (6, 28) | (6, 28) | (6, 29) | (6, 29) | (6, 29) | (6, 29) | (6, 29) | (6, 30) | |
| (6, 30) | (6, 30) | (6, 30) | (6, 30) | (6, 30) | (7, 1) | (7, 1) | (7, 1) | (7, 2) | (7, 2) | |
| (7, 2) | (7, 2) | (7, 4) | (7, 4) | (7, 4) | (7, 4) | (7, 4) | (7, 4) | (7, 5) | (7, 5) | |
| (7, 5) | (7, 6) | (7, 6) | (7, 6) | (7, 7) | (7, 7) | (7, 7) | (7, 7) | (7, 7) | (7, 8) | |
| (7, 8) | (7, 8) | (7, 9) | (7, 12) | (7, 12) | (7, 12) | (7, 13) | (7, 13) | (7, 13) | (7, 13) | |
| (7, 13) | (7, 13) | (7, 13) | (7, 14) | (7, 14) | (7, 15) | (7, 15) | (7, 15) | (7, 15) | (7, 16) | |
| (7, 16) | (7, 17) | (7, 17) | (7, 17) | (7, 18) | (7, 18) | (7, 19) | (7, 19) | (7, 19) | (7, 20) | |
| (7, 20) | (7, 20) | (7, 20) | (7, 20) | (7, 20) | (7, 21) | (7, 21) | (7, 21) | (7, 21) | (7, 23) | |
| (7, 23) | (7, 24) | (7, 24) | (7, 24) | (7, 25) | (7, 25) | (7, 25) | (7, 25) | (7, 26) | (7, 26) | |
| (7, 26) | (7, 27) | (7, 28) | (7, 28) | (7, 30) | (7, 30) | (7, 30) | (7, 30) | (7, 31) | (7, 31) | |
| (8, 1) | (8, 3) | (8, 3) | (8, 3) | (8, 3) | (8, 3) | (8, 3) | (8, 4) | (8, 4) | (8, 4) | |
| (8, 4) | (8, 5) | (8, 5) | (8, 5) | (8, 5) | (8, 7) | (8, 7) | (8, 7) | (8, 7) | (8, 8) | |
| (8, 8) | (8, 9) | (8, 9) | (8, 9) | (8, 10) | (8, 10) | (8, 10) | (8, 10) | (8, 11) | (8, 12) | |
| (8, 13) | (8, 13) | (8, 13) | (8, 14) | (8, 15) | (8, 15) | (8, 15) | (8, 15) | (8, 16) | (8, 16) | |
| (8, 17) | (8, 18) | (8, 18) | (8, 19) | (8, 20) | (8, 20) | (8, 20) | (8, 20) | (8, 20) | (8, 20) | |
| (8, 21) | (8, 22) | (8, 22) | (8, 22) | (8, 22) | (8, 22) | (8, 23) | (8, 23) | (8, 24) | (8, 24) | |
| (8, 24) | (8, 25) | (8, 25) | (8, 26) | (8, 26) | (8, 26) | (8, 26) | (8, 27) | (8, 27) | (8, 28) | |
| (8, 29) | (8, 30) | (8, 30) | (8, 30) | (8, 30) | (8, 31) | (8, 31) | (8, 31) | (8, 31) | (9, 2) | |
| (9, 3) | (9, 3) | (9, 4) | (9, 5) | (9, 5) | (9, 5) | (9, 6) | (9, 6) | (9, 6) | (9, 7) | |
| (9, 8) | (9, 9) | (9, 9) | (9, 9) | (9, 10) | (9, 11) | (9, 12) | (9, 12) | (9, 13) | (9, 14) | |
| (9, 14) | (9, 15) | (9, 15) | (9, 16) | (9, 16) | (9, 16) | (9, 17) | (9, 18) | (9, 18) | (9, 18) | |
| (9, 18) | (9, 19) | (9, 19) | (9, 20) | (9, 20) | (9, 20) | (9, 20) | (9, 21) | (9, 21) | (9, 22) | |
| (9, 22) | (9, 23) | (9, 23) | (9, 24) | (9, 24) | (9, 24) | (9, 24) | (9, 24) | (9, 25) | (9, 25) | |
| (9, 25) | (9, 26) | (9, 28) | (9, 28) | (9, 28) | (9, 29) | (9, 30) | (9, 30) | (9, 30) | (9, 30) | |
| (10, 1) | (10, 2) | (10, 2) | (10, 3) | (10, 3) | (10, 3) | (10, 3) | (10, 3) | (10, 4) | (10, 5) | |
| (10, 5) | (10, 5) | (10, 6) | (10, 7) | (10, 7) | (10, 8) | (10, 8) | (10, 8) | (10, 8) | (10, 9) | |
| (10, 9) | (10, 9) | (10, 9) | (10, 9) | (10, 10) | (10, 10) | (10, 10) | (10, 10) | (10, 11) | (10, 12) | |
| (10, 12) | (10, 13) | (10, 13) | (10, 13) | (10, 13) | (10, 13) | (10, 13) | (10, 13) | (10, 13) | (10, 14) | |
| (10, 14) | (10, 15) | (10, 15) | (10, 15) | (10, 17) | (10, 17) | (10, 18) | (10, 20) | (10, 21) | (10, 22) | |
| (10, 22) | (10, 22) | (10, 23) | (10, 23) | (10, 23) | (10, 24) | (10, 24) | (10, 24) | (10, 25) | (10, 25) | |
| (10, 26) | (10, 26) | (10, 28) | (10, 30) | (10, 30) | (10, 30) | (10, 30) | (10, 31) | (10, 31) | (10, 31) | |
| (11, 1) | (11, 1) | (11, 2) | (11, 3) | (11, 3) | (11, 4) | (11, 5) | (11, 5) | (11, 5) | (11, 6) | |
| (11, 8) | (11, 8) | (11, 9) | (11, 9) | (11, 9) | (11, 9) | (11, 9) | (11, 9) | (11, 10) | (11, 10) | |
| (11, 10) | (11, 10) | (11, 11) | (11, 12) | (11, 14) | (11, 14) | (11, 15) | (11, 16) | (11, 16) | (11, 16) | |
| (11, 16) | (11, 16) | (11, 16) | (11, 16) | (11, 18) | (11, 19) | (11, 20) | (11, 20) | (11, 21) | (11, 21) | |
| (11, 21) | (11, 22) | (11, 22) | (11, 23) | (11, 23) | (11, 24) | (11, 24) | (11, 24) | (11, 25) | (11, 26) | |
| (11, 26) | (11, 26) | (11, 26) | (11, 27) | (11, 27) | (11, 27) | (11, 28) | (11, 29) | (11, 29) | (11, 29) | |
| (11, 29) | (11, 30) | (11, 30) | (11, 30) | (12, 1) | (12, 1) | (12, 2) | (12, 2) | (12, 2) | (12, 3) | |
| (12, 4) | (12, 5) | (12, 5) | (12, 5) | (12, 5) | (12, 5) | (12, 6) | (12, 6) | (12, 6) | (12, 6) | |
| (12, 7) | (12, 7) | (12, 7) | (12, 7) | (12, 7) | (12, 8) | (12, 8) | (12, 8) | (12, 9) | (12, 9) | |
| (12, 9) | (12, 9) | (12, 9) | (12, 9) | (12, 9) | (12, 10) | (12, 10) | (12, 10) | (12, 12) | (12, 12) | |
| (12, 12) | (12, 13) | (12, 13) | (12, 14) | (12, 14) | (12, 14) | (12, 14) | (12, 15) | (12, 15) | (12, 15) | |
| (12, 15) | (12, 15) | (12, 16) | (12, 16) | (12, 17) | (12, 17) | (12, 17) | (12, 17) | (12, 18) | (12, 18) | |
| (12, 18) | (12, 18) | (12, 18) | (12, 19) | (12, 19) | (12, 20) | (12, 21) | (12, 22) | (12, 22) | (12, 23) | |
| (12, 23) | (12, 23) | (12, 23) | (12, 23) | (12, 24) | (12, 24) | (12, 24) | (12, 24) | (12, 25) | (12, 25) | |
| (12, 26) | (12, 26) | (12, 26) | (12, 26) | (12, 27) | (12, 27) | (12, 27) | (12, 28) | (12, 28) | (12, 29) | |
| (12, 29) | (12, 29) | (12, 29) | (12, 31) | (12, 31) | (12, 31) |
(Analyze the data according to your original test plan. Draw conclusions based off of that plan.)
| Zodiac Sign | Expected Ratio | Observed Ratio | |
| Capricorn | 0.082 [4] | 0.073 | |
| Aquarius | 0.082 | 0.087 | |
| Pieces | 0.082 | 0.087 | |
| Aries | 0.085 | 0.082 | |
| Taurus | 0.085 | 0.094 | |
| Gemini [5] | 0.088 | 0.103 | |
| Cancer | 0.085 | 0.088 | |
| Leo | 0.085 | 0.074 | |
| Virgo | 0.082 | 0.081 | |
| Libra | 0.085 | 0.070 | |
| Scorpio | 0.079 | 0.096 | |
| Sagittarius | 0.079 | 0.066 |
[4]All figures here have been rounded to three decimal points for display purposes.
[5]In the image, the word in yellow is "Gemini"; my apologies for it being hard to read.
The distance between the expected and observed incidence of occurrence is 0.094 [6]. Next, to establish the p-value associated with this number, 100000 simulations were run in which 876 birthdays were randomly distributed through the calendar year, and the error values for each one was calculated. In 26.74% of the simulations, the error value was higher than the observed error value. Since 0.2674 > 0.05, the null hypothesis is not rejected by this experiment.
[6]The fact that the observed distribution is not even may lead an empiricist to conclude that there is something going on. This conclusion is not necessarily wrong; empirical science does not prove negative statements. Instead, we merely cannot conclude that it's right from this experiment. It's very easy to find patterns that empiricism does not reveal; in running this experiment, I had the subjective observation that one of the signs with fewer observed friends' birthdays (Sagittarius) also included a number of frenemies, and that two of my exes share a birthday along with another good friend. None of these observations are supported by this evidence.
For your viewing pleasure, I have rendered the calendar year with all my friends' birthdays, delimiting it by month and zodiac sign.